
Unveiling Order in Cosmic Chaos: A Revolutionary Look at the Three-Body Problem
For centuries, the Three-Body Problem has perplexed mathematicians and physicists, describing the seemingly unpredictable gravitational interactions among three massive objects in space. Traditionally deemed unsolvable due to its chaotic nature, this celestial conundrum is now being re-examined. A groundbreaking study by Dr. Alessandro Alberto Trani from the University of Copenhagen’s Niels Bohr Institute reveals that these interactions can exhibit surprising regularities, challenging long-held beliefs about cosmic chaos. His research was recently published in the journal Astronomy & Astrophysics.
Recently, we had the privilege to speak with Dr. Trani. During our conversation, he shared his research and insights from his new findings about this complex celestial Three-Body Problem. His work uncovers “islands of regularity” within the chaotic dance of celestial bodies, suggesting that the outcomes are not entirely random but often depend predictably on initial conditions like positions, velocities, and angles of approach.
Join us as we delve into Dr. Trani’s revolutionary research, exploring how this discovery could enhance our understanding of gravitational waves and the fundamental forces shaping our universe. Please enjoy our exclusive interview with Dr. Alessandro Alberto Trani.

Q: Could you explain the traditional understanding of the Three-Body Problem and why it has been considered unsolvable in mathematics and theoretical physics?
A: The Three-Body Problem is a very ancient issue that originated with Isaac Newton and has been explored by many foundational figures in physics, astronomy, and astrophysics. The concept begins with Newton’s successful solution to the Two-Body Problem, which describes how two objects interact under gravity. This problem is considered an integrable system, meaning we can find exact analytical solutions. Given the initial positions and velocities of the two bodies, we can predict their future motions precisely.
However, the Three-Body Problem is fundamentally different and not integrable. It was first intensively studied to understand the motion of the Moon, as the Earth, Moon, and Sun form a three-body system—the closest such example to us. Early scientists realized they couldn’t accurately predict the Moon’s orbit, particularly its precession and the advancement of its perigee (the point in its orbit closest to Earth).
Henri Poincaré, around the end of the 19th century, discovered that the Three-Body Problem exhibits chaotic behavior. His work demonstrated that small differences in initial conditions could lead to vastly different outcomes, making long-term predictions impossible. Since Poincaré’s findings, researchers have relied on numerical methods—computer simulations—to study the problem, as analytical solutions are unattainable.
In recent times, we’ve begun using statistical approaches to navigate the chaos inherent in the Three-Body Problem. By treating the system statistically, we hoped to predict outcomes based on probabilities rather than exact solutions. Until my recent work, it was generally accepted that the Three-Body Problem was purely chaotic, and we leveraged that chaos using statistical theories to predict interaction outcomes.
However, my research has revealed that the Three-Body Problem is not solely chaotic; it is actually a mix of chaos and regularity. This means that there are regions where the system behaves predictably amidst the chaos. This discovery complicates our understanding and challenges the effectiveness of purely statistical methods, as the presence of regularity affects our ability to make accurate predictions. It suggests that we need to develop new approaches that account for both the chaotic and regular aspects of the Three-Body Problem.
Q: What initially drew you to study this complex problem, and how does it relate to your broader research interests?
A: Curiosity was the main driving force that drew me to this complex problem. I approached it from an astrophysical perspective because I’m an astrophysicist, not a mathematician. My goal was to understand three-body interactions and how we can use them to solve astrophysical problems.
For example, just as early scientists applied the three-body problem to understand the Moon’s motion influenced by the Earth and the Sun, we apply it to black holes and gravitational waves. Over the past decade, we’ve begun detecting gravitational waves here on Earth, which has opened a new window into the universe. However, we still don’t fully understand where these gravitational waves originate.
One possible explanation is that they come from black holes—the remnants of massive stars—that encounter each other, emit gravitational wave energy, and eventually coalesce. We can detect these events with our instruments on Earth. We know that such three-body interactions are likely to occur at the centers of giant ensembles of stars known as star clusters. These clusters exist throughout the universe, and their evolution is significantly influenced by three-body interactions at their cores.
While we can’t directly observe interactions between black holes because they don’t emit light (they’re black, after all), we can study the gravitational waves they produce. Understanding the three-body problem helps us interpret these gravitational waves and provides insights into the dynamics within star clusters. This research bridges my interest in astrophysics with the fundamental challenges posed by the three-body problem.
Q: Your research suggests the existence of ‘isles of regularity’ amidst the chaos of three-body interactions. Can you describe what these are and how they challenge the conventional wisdom about the Three-Body Problem?
A: Certainly! Traditionally, the Three-Body Problem has been considered entirely chaotic, meaning that predicting the long-term behavior of three gravitationally interacting bodies is virtually impossible due to extreme sensitivity to initial conditions. Small differences at the start can lead to vastly different outcomes, making the system unpredictable.
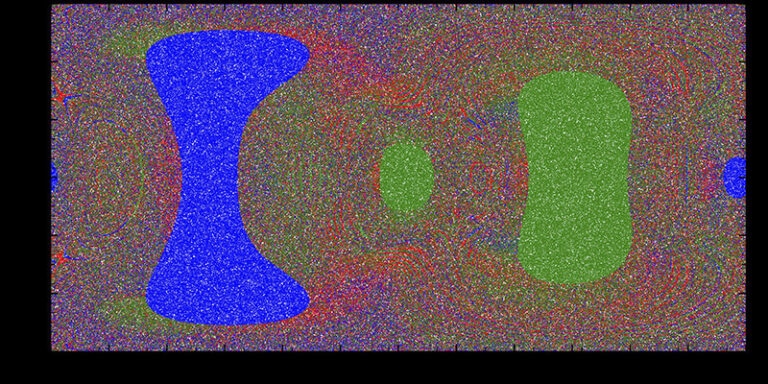
However, my research has uncovered what we call “isles of regularity” within this chaotic landscape. These are regions in the map of possible initial conditions where the system behaves in a predictable, regular manner. To visualize this, imagine a diagram where each point represents a specific initial configuration of the three bodies, and each point is colored based on the outcome of the interaction—for instance, which body gets ejected from the system.
In a purely chaotic system, all points should have a random color, resulting in an indistinguishable mix of the three colors—because even initial configurations that are very close result in a different outcome. However, we observe four large, distinctly colored regions—represented in blue and green—that stand out against the surrounding chaotic background. In these “isles of regularity”, even if we change the initial configuration, the final outcome is always the same.
These “isles” correspond to scenarios where the three-body system breaks apart very quickly, and one of the bodies is expelled rapidly. Because the interaction is so brief, there isn’t sufficient time for chaotic behavior to develop, resulting in a predictable outcome.
This discovery challenges the conventional wisdom by demonstrating that the Three-Body Problem is not purely chaotic; instead, it exhibits a mix of chaos and regularity. The existence of these regular regions means that, under certain initial conditions, we can predict the outcome of three-body interactions with greater confidence.
To put it in perspective, consider our own solar system. While it’s a complex system with many bodies, it is relatively stable over long periods. The Earth-Moon-Sun system, for example, doesn’t exhibit the kind of chaos we see in typical three-body problems because the Moon remains in a stable orbit around Earth. If we were to significantly alter the Moon’s orbit—say, by giving it an extra push—it could become unstable, potentially leading to chaotic behavior where the Moon might eventually escape Earth’s gravitational pull. This would resemble the unstable scenarios studied in the Three-Body Problem.
In summary, the “isles of regularity” reveal that predictability exists within the Three-Body Problem under specific conditions. This finding not only challenges long-held assumptions but also enhances our ability to model and understand complex gravitational interactions in astrophysics.
Q: How do the initial positions, velocities, and angles of approach influence these predictable patterns?
A: The initial positions, velocities, and angles of approach play a crucial role in influencing the predictable patterns observed within the “isles of regularity” in three-body interactions. We know that there is a precise mapping between these initial conditions and the final outcomes of the system. This means that specific starting configurations lead to specific results, especially within these regular regions.
However, we haven’t yet fully determined what this precise mapping looks like. While we recognize that a definitive link exists in these islands of regularity, we currently lack a comprehensive theory to explain and predict the outcomes based on initial conditions. In contrast, for the chaotic regions—the “chaotic sea”—we can predict outcomes statistically because chaos allows us to apply statistical methods effectively over many simulations.
The challenge with the regular islands is that they don’t conform neatly to statistical analysis; their predictability isn’t random but also isn’t fully understood. One potential approach to overcoming this hurdle is to use machine learning and artificial intelligence to predict these regions and their outcomes. AI could help identify patterns and mappings that are not immediately apparent through traditional methods.
However, as astrophysicists, we aim to develop physical models that we can understand and interpret. While AI can provide predictions, it might not offer the underlying physical insights we’re seeking. Our goal is to formulate a theory grounded in physics that explains how initial conditions lead to specific outcomes in these regular regions, enhancing our overall understanding of three-body interactions.
Q: You developed your own software program, Tsunami, to run millions of simulations. What were the key features of this program, and why was it necessary to create a new tool for your research?
A: Yes, that’s correct. Simulating this kind of interaction is very challenging with standard numerical integrators because these interactions are highly chaotic. Due to their chaotic nature, even a very small error during the simulation can propagate and become exponentially amplified over time through numerical errors. This amplification can introduce artificial chaos into the simulation results, something we refer to as “numerical chaos.” It’s not a natural part of the physical system but an artifact introduced by the numerical methods themselves.
Therefore, it’s crucial to have simulation software that performs with very high accuracy to minimize these numerical errors. That’s why I developed my own code called Tsunami. I created it while I was in Japan, and the name is fitting because my code includes modeling of tidal interactions—tsunami meaning “tidal wave” in Japanese, even though it is a phenomenon unrelated to tides. Developing Tsunami allowed me to run highly accurate simulations necessary for my research into three-body interactions and to avoid the pitfalls of numerical chaos that could compromise the results.
Q: Can you walk us through the process of setting up and interpreting these simulations? What parameters did you vary, and how did you manage the vast amount of data generated?
A: The Three-Body Problem is inherently a highly multidimensional issue—specifically, it’s an 18-dimensional problem. This comes from the fact that we have three particles in the system. Each particle has three positional coordinates and three velocity components, totaling six variables per particle. So, 3 particles×6 variables=18 dimensions3.
Visualizing or analyzing an 18-dimensional space directly is extremely challenging. To make the problem more tractable, I focused on a two-dimensional slice of this multidimensional space. This slice included two key parameters:
*The Orbital Phase of the Binary System at the Beginning: This is the position of the two-body subsystem (the binary) along its orbit at the start of the simulation.
*The Inclination Angle: This is the angle between the plane of the binary’s orbit and the line along which the third body approaches.
By selecting these two angles as initial conditions, I could represent the system in a two-dimensional plot. In this representation, the X-axis ranged from 0∘ to 360∘ (covering the full orbital phase), and the Y-axis ranged from −90∘ to 90∘ (covering all possible inclinations). This approach made it much easier to visualize the parameter space and to observe patterns or “isles of regularity” within the chaotic dynamics.
In addition to these two parameters, I also explored variations in:
*Initial Velocities: Adjusting the speed at which the third body approaches the binary.
*Impact Parameters: Changing how close the third body comes to the binary system during its approach.
Interestingly, I found that varying these additional parameters did not significantly alter the overall results. This suggests that the phenomena we observed are robust across different initial conditions.
Regarding the computational aspect, managing the vast amount of data generated from millions of simulations required efficient data handling strategies. I developed my own simulation code, Tsunami, which is optimized for accuracy and computational efficiency. Tsunami minimizes numerical errors that can accumulate over time in chaotic systems, which is crucial for maintaining the integrity of the simulations.
The binary system in these simulations consists of two bodies already bound in orbit around each other—they form a stable binary. When a third body approaches, it can perturb this system. Depending on the initial conditions, the interaction can lead to various outcomes, such as one body being ejected or the formation of a new binary configuration. By focusing on specific slices of the parameter space, we can better understand these interactions and the conditions that lead to predictable versus chaotic behavior.
In summary, by reducing the complexity of the problem through careful selection of parameters and by using specialized software to handle computations and data management, we were able to effectively set up, run, and interpret a vast number of simulations to study the Three-Body Problem in greater detail.
Q: Currently, the most popular show on Netflix is the science fiction series “The Three-Body Problem,” based on a Chinese novel series by Liu Cixin. Did this film inspire your research?
A: The movie is quite interesting, but it’s important to remember that it’s a work of fiction. My research isn’t influenced by the film, and my results don’t alter any aspects of its narrative.
In fact, the scenario presented in the movie is more complex than the traditional Three-Body Problem; it’s actually a Four-Body Problem because it involves three stars and one planet. This makes it significantly more complicated. I attempted to run simulations of such a system and found it very challenging to produce a stable planetary orbit like the one depicted in the movie.
My findings indicate that a planet in such a system can either have a regular (predictable) orbit or a chaotic one, but it cannot be both simultaneously. Additionally, a system cannot transition from chaos to regularity; if it’s chaotic, it will remain chaotic, and if it’s regular, it will stay regular. There are distinct regions in the parameter space where the system behaves either chaotically or regularly, and these regions are quite well separated.
A planet that exists in a regular region will always exhibit regular behavior, while one in a chaotic region will display chaotic dynamics. Within chaotic regions, there might be brief periods of regularity, but these typically don’t last long, and we can’t predict how long they will persist.
When conducting my simulations, I consider various factors, such as the masses of the bodies (whether they are black holes, stars, or planets) and the distances between them. There are many variations to account for to accurately simulate these complex interactions.
The advantage is that if we only consider Newtonian gravity—without incorporating the effects of Einstein’s General Relativity—we can rescale the simulations to any scale. For instance, I might model the three bodies as black holes, but by adjusting the masses and distances, they could represent planets or supermassive black holes millions of times the mass of the sun that reside at the centers of galaxies. In this way, the Three-Body Problem is very general and applicable to various astrophysical scenarios.
Q: How might your findings impact our understanding of gravitational waves and the interactions between massive celestial bodies like black holes?
A: My findings are very important because they have practical applications in astrophysics, not just in mathematical theory. We use the statistical theories of the Three-Body Problem to make predictions about what we can observe with gravitational wave observatories. My research shows that the regular regions within the Three-Body Problem are crucial because many binary systems that form in these regions involve two black holes coming very close together. This close approach increases the likelihood that they will merge into a single black hole, producing gravitational waves in the process.
These kinds of events happen to be three times more frequent in regular regions than in chaotic ones. Therefore, the regular regions may be key to explaining the gravitational wave events we observe today. Understanding these regular interactions enhances our ability to predict and interpret gravitational wave signals resulting from black hole mergers and other massive celestial interactions.
Q: In what ways could this research contribute to improving astrophysical models and our comprehension of fundamental forces like gravity?
A: This research can significantly enhance our understanding of how gravitational interactions occur at the centers of star clusters. By studying three-body interactions, we can learn how these gravitational encounters lead to observable gravitational waves. This knowledge allows us to refine astrophysical models that predict such events, improving their accuracy and reliability.
Moreover, by understanding these intricate gravitational processes, we deepen our comprehension of fundamental forces like gravity. This not only helps us unravel the mysteries of the universe but also provides insights into our own place within it. In essence, as we learn more about the universe’s workings, we gain a better understanding of ourselves and the fundamental laws that govern everything around us.
Q: You mentioned that the discovery of regularities complicates statistical probability calculations. Can you elaborate on this challenge and how it affects predictions in astrophysics?
A: In chaotic systems like the traditional Three-Body Problem, predicting individual outcomes is extremely difficult due to the sensitive dependence on initial conditions. However, chaos has a property where the specifics of past states become less relevant over time. This allows us to use statistical theories to predict the overall behavior of many chaotic systems collectively. We can make probabilistic predictions about outcomes because the chaotic nature effectively “averages out” individual variations.
The challenge arises with the discovery that the Three-Body Problem isn’t entirely chaotic—it also contains regions of regularity. In these regular regions, the system’s behavior is predictable and strongly dependent on initial conditions. Regular interactions do not lose the memory of their starting configurations, unlike chaotic ones.
As a result, the statistical methods we use for chaotic systems, which rely on the assumption that past details become irrelevant, do not apply to regular interactions.
This complicates our calculations because we can no longer use a single statistical framework to predict outcomes across the entire system. We must now distinguish between chaotic and regular regions:
*For Chaotic Regions: We can continue to use statistical theories to make probabilistic predictions.
*For Regular Regions: We need deterministic methods or new models that account for the predictable nature of these interactions.
In astrophysics, this means that our ability to predict phenomena like gravitational wave events or the outcomes of three-body interactions becomes more complex. We have to account for both the chaotic and regular behaviors, developing new approaches for each. The presence of regularities forces us to refine our models and perhaps integrate different theoretical frameworks to accurately predict astrophysical events.
Q: What approaches are you considering to integrate statistical methods with high-precision numerical calculations to address this issue?
A: It’s a very complex challenge. At the moment, the only feasible approach is to run millions of numerical simulations. By doing so, we can use some sort of metric for chaos to identify and exclude the regular islands, focusing only on the chaotic interactions. This allows us to compare chaos and regularity with the predictions of statistical theories.
The difficulty arises when we try to integrate deterministic mechanical theories—which are not statistical—to predict the regular regions. It’s challenging to mix these with statistical methods correctly. For example, I find that about 37%—more than one-third—of the parameter space is regular. However, if we change the initial positions of the bodies, this fraction can vary significantly—it might increase to 80% or decrease to 20%. This variability makes it very difficult to know beforehand where chaos and regularity will occur without running the simulations.
Therefore, the current approach is first to find a way not just to predict these regular regions but also to determine a priori where chaos and regularity exist. Right now, we don’t have this capability. We can only rely on numerical simulations to map out these regions.
Q: Have you considered dark matter in your calculations as one of the variables?
A: Yes, we have considered dark matter in our calculations, particularly because there’s still a possibility that dark matter may be composed of black holes. If dark matter is made up of what we call primordial black holes—black holes that formed at the beginning of the universe—then the interactions we study could indeed represent the interactions of these black holes that constitute dark matter.
However, in the more standard conceptual picture, dark matter is thought to consist of particles that do not interact closely with each other through gravitational forces like black holes do. Unfortunately, this means that traditional dark matter particles wouldn’t engage in the kind of three-body interactions we model in our simulations. But if dark matter were composed of black holes, as some theories suggest, then our work would be directly applicable to understanding those interactions.
Q: Do you foresee applications of your research in understanding complex systems or chaos theory in other disciplines?
A: Yes, it’s a possibility. The Three-Body Problem is an example of a natural system that, despite its simplicity, exhibits complex chaotic behavior. Similarly, other systems like the double pendulum are simple yet display chaotic dynamics. The double pendulum shares many features with the Three-Body Problem, such as the mixture of regular and chaotic regions.
The nature of this interplay between regularity and chaos is likely applicable to many other systems beyond the Three-Body Problem. My next step is to understand how to predict these regular regions, either by developing a deterministic theory or by using machine learning and artificial intelligence to comprehend the regularity. By doing so, we can make better predictions about phenomena in the universe, such as gravitational waves.
It’s a significant project that involves not just me but also collaborators around the world.
Q: How critical were advancements in computational power and software development to achieving your results?
A: That’s a very good question. Advancements in computational power and software development were absolutely critical to achieving my results. Our research was enabled by these technological and software improvements because, until recently, nobody realized that the N-body problem—specifically the Three-Body Problem—exhibited both regular and chaotic behavior simultaneously. We always assumed it was only chaotic.
One of the main reasons for this misconception was the limitations of numerical simulations in the past. We didn’t have enough simulations, and those we did have were not very accurate due to insufficient computational resources. Without the necessary computational power and precise software, it was impossible to perform the extensive simulations required to reveal the regularities within the chaos.
The advancements in computational capabilities allowed us to run millions of high-precision simulations using specialized software. This enabled us to identify the “isles of regularity” amidst the chaotic behavior of three-body interactions. So, the improvements in computational power and software were essential for conducting this kind of detailed study and achieving our groundbreaking results.
Q: The interplay between chaos and order has long fascinated philosophers and scientists alike. How do your discoveries contribute to this ongoing discourse?
A: In a sense, my work suggests that there isn’t necessarily a conflict between chaos and order; instead, each defines the absence of the other. One of the insights from my research is that when you examine a chaotic region and zoom in closer—looking at finer and finer details—you begin to see that chaos actually contains pockets of regularity.
So perhaps chaos is a matter of perspective or scale. What appears chaotic from a distance may reveal small “isles of regularity” when viewed up close. Even within a vast sea of chaos, there are patterns and orderly structures that emerge upon closer inspection. This interplay implies that chaos and order are interconnected, and understanding one requires acknowledging the presence of the other.
Q: What are your thoughts on the deterministic nature of the universe in light of finding regularities within chaotic systems?
A: It’s very interesting because regularity and chaos are both deterministic in nature. However, chaos acts as a kind of disconnect between the deterministic past and the deterministic future because it makes predictions practically impossible. Theoretically, it’s predictable, as any deterministic system, but in practice, it’s not because it requires perfect knowledge of the initial state of the system, like positions and velocities and masses.
What makes this more complex is that the universe also includes effects that are not deterministic, like quantum effects. In my research on the three-body problem, I found that if you zoom in enough, there comes a point where even the tiniest change in the initial configuration is smaller than the smallest quantum scale we can measure—what we call the Planck length.
The Planck length is a scale below which we cannot measure anything with certainty. Yet, I’ve found that even a change smaller than this, in terms of the distance between objects, can lead to vastly different outcomes in how the system interacts. So, while the system is still deterministic, we would be unable to measure those initial differences accurately enough to predict future behavior.
In a sense, the universe is deterministic in principle, but in practice, it feels like it is not. Because of chaos, “the present determines the future, but the approximate present does not approximately determine the future”. This unpredictability might offer some explanation for how the universe appears deterministic yet still allows for freedom of choice—since we cannot precisely connect the past with the future, due to our limited knowledge of the past.
Q: Is there anything else you’d like to share about your research or its significance that we haven’t covered?
A: Yes, I’d like to add that I truly enjoyed this research because it was a deeply personal project for me. I found great satisfaction in working on a problem that is over 300 years old but also has very modern applications. I believe this is the best kind of science—it combines fundamental, historical inquiry with contemporary relevance. By bridging the gap between old and new science, we can apply timeless principles to understand current phenomena in the universe. This fusion of the classical and the modern enhances our overall comprehension and makes the research both meaningful and exciting.
Q: How do you hope your work will inspire future generations of scientists and mathematicians?
A: I hope that my work will attract more young people to this field by demonstrating that there is something deeply appealing about tackling problems that may be very old. They might see and understand that an old problem can still hold significant relevance today.
I believe this research can help make young people aware that revisiting classical problems is valuable—not just following the latest trends or developments, thinking those are the only interesting areas. In fact, old problems can lead to new solutions for contemporary challenges. By showing that longstanding questions still have much to offer, I hope to inspire future generations to explore both the historical and modern aspects of science and mathematics.
In conclusion, Dr. Alessandro Alberto Trani’s groundbreaking work not only challenges centuries-old perceptions of the Three-Body Problem but also opens new avenues for understanding the complexities of our universe. By revealing the hidden “isles of regularity” within chaotic gravitational interactions, his research bridges the gap between classical physics and modern astrophysics. This discovery holds the potential to revolutionize our approach to modeling cosmic phenomena, enhancing our grasp of gravitational waves, black hole mergers, and the fundamental forces that govern celestial mechanics. As we continue to explore the cosmos, insights like Trani’s remind us that even amidst apparent chaos, underlying patterns await discovery, enriching our quest to comprehend the vastness of space.
